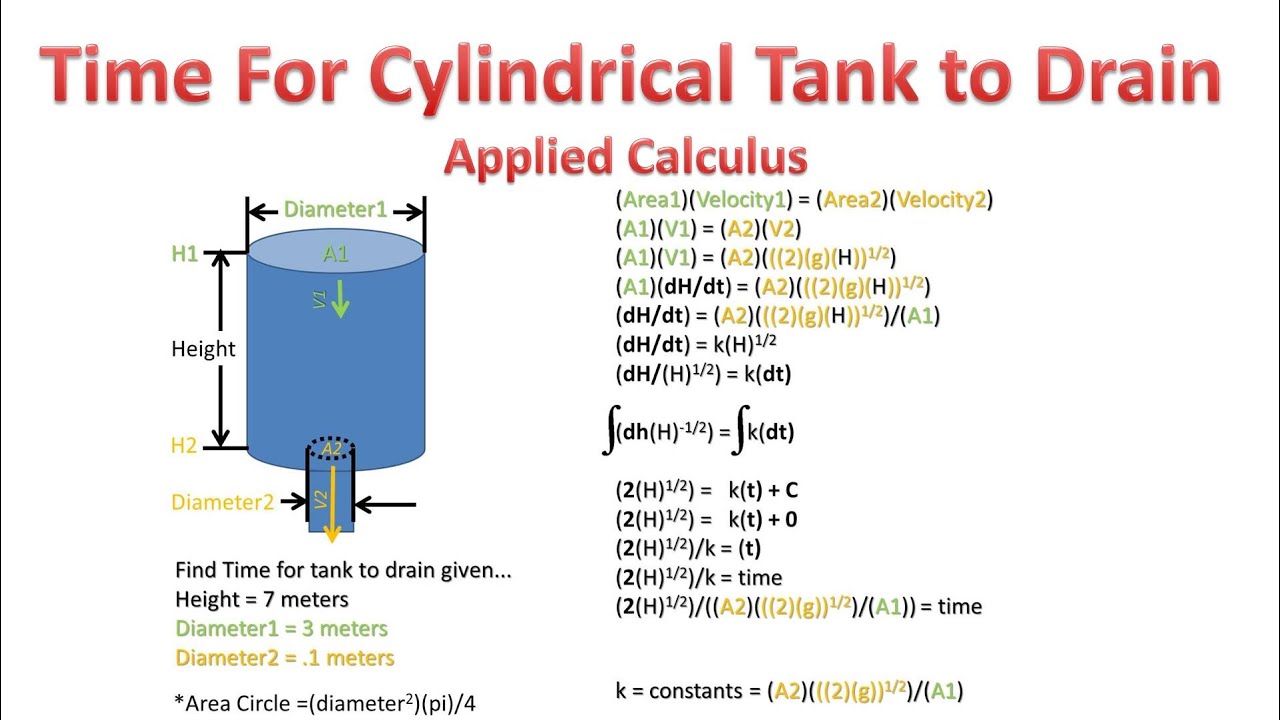
In this problem we have a cylindrical tank that is open at the top and filled with water. We want to find the time it will take for this to drain assuming an ideal fluid (ignore friction, viscosity , turbulence etc) We will be reffering to the top of the tank as point 1 and this has a area1 and height1. We will also be defining point 2 as the point where the fluid is leaving the tank this has an area 2 and a height 2 tied to it.
I have listed all of the values for the diameters and height we will be working with. How do we go about solving this one?
Well recall from the piston displacing water video that the Q or flow rate is conserved. So the same volume that the tank is lowering by is the same volume that is leaving the tank. This means we can tak area 1 time velocity 1 and set it equal to area 2 time velocity 2. Cross sectional area times velocity of fluid through that cross sectional area is the flow rate at that point.
Recall from the torricellis law video that the velocity of water coming out of the bottom of a tank is the same as a free falling water droplet that is dropped from the water line of the tank. (this is under ideal circumstances) So the equation for the velocity2 the velocity at the hole in the bottom of the tank is the square root of 2 times g times the height from the water line to the hole in the bottom. I will be notating square roots as to the ½ power which is equavelent to the square root.
Now we need to define velocity 1 or the rate at which the fluid in the tank lowers over time. This is really just the delta in height or change in height divided by the delta of time or change in time. The change in displacement over the change in time is the definition of velocity. We can now plug D H over D t into the equation where velocity once was.
For our next step we need to get everything to one side except for the DH over DT. Then we will group all of the values that remain constant and set that value equal to K. then replace all of those values with the letter k in the original equation. We get dh over dt is equal to k times square root of the height of the water in the tank at initial condition.
Next we will divide both sides the the square root of the height.
Now we can integrate both sides of the equation with respect to the variable that is changing on either sides. Recall that raising something to the negative power is the same as dividing by that value to that power I did this to height.
We use a combination of the power rule and the constant rule… which for your reference are listed on this slide. We are left with 2 times square root of h being equal to k times t plus c
Our constant in this case will be 0
Now if we divide both sides by k we get the equation for the time for the fluid to drain from the tank
We can now plug in what our k constant value is equal to.
And plug in all of our values that I listed at the beginning of the problem
We get a time of 1,075 seconds which equals
17.9 minutes to drain this tank. Keep in mind this is an ideal example so in reality it will probably drain slower.
Here is the video tally for the closed poll that I put on my community tab this adds one more video to applied calculus.

Disclaimer
These videos are intended for educational purposes only (students trying to pass a class) If you design or build something based off of these videos you do so at your own risk. I am not a professional engineer and this should not be considered engineering advice. Consult an engineer if you feel you may put someone at risk.

свернуть