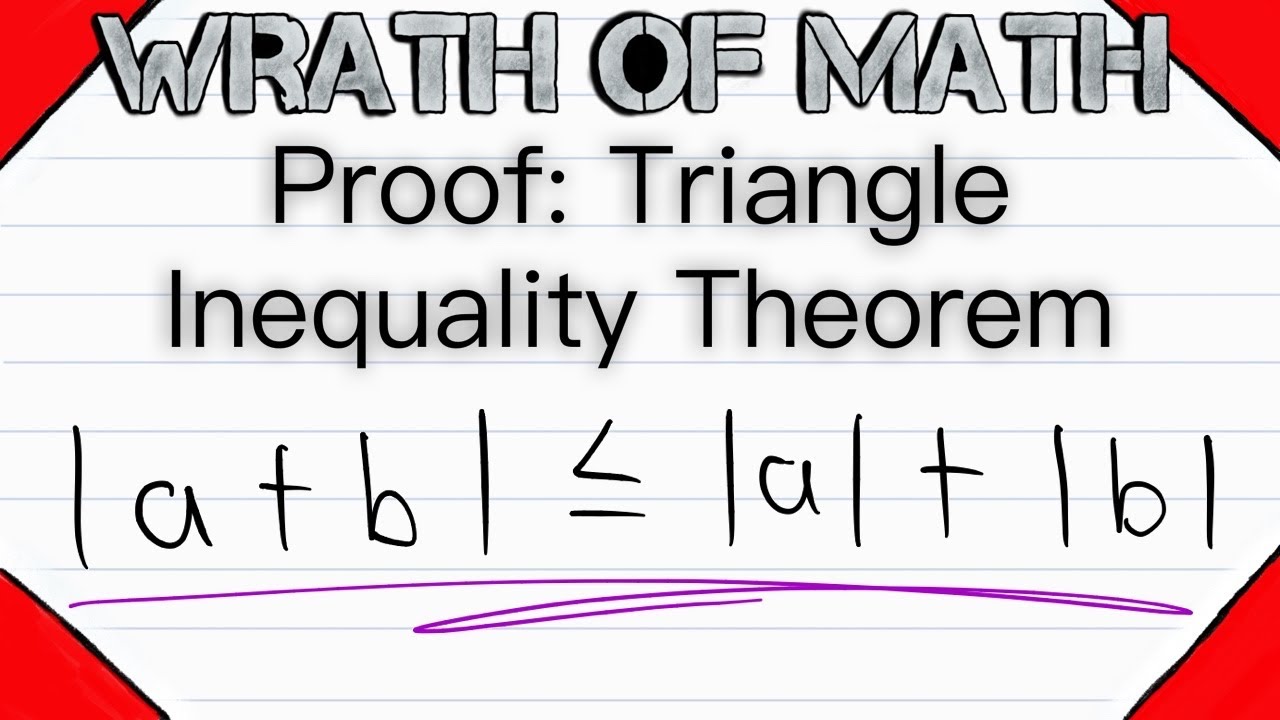
The absolute value of a sum is less than or equal to the sum of the absolute values for any two real numbers. That is: |a+b| is less than or equal to |a|+|b|. This is called the triangle inequality. It's very useful in real analysis and we'll prove it in today's lesson! The name of the theorem is explained a little below.
If we considered a triangle made of vectors x, y, and z, with x and z starting at the origin, then the vector z is the vector x+y, and thus the theorem says this third side (z) of the triangle must be no greater than the sum of the other two sides: |z| is less than or equal to |x| + |y| and since the vector z is the vector x+y we have |x+y| is less than or equal to |x| + |y|. Speaking strictly in terms of geometry, we'd often use a strictly less than inequality for this theorem because the equality case describes a "degenerate" triangle, where the "triangle" is just a line. However, the "less than or equal to" inequality is perfectly valid and reasonable for real numbers.
◆ Donate on PayPal: [ Ссылка ]
◆ Support Wrath of Math on Patreon: [ Ссылка ]
I hope you find this video helpful, and be sure to ask any questions down in the comments!
********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.
Vallow Bandcamp: [ Ссылка ]
Vallow Spotify: [ Ссылка ]
Vallow SoundCloud: [ Ссылка ]
********************************************************************
+WRATH OF MATH+
Follow Wrath of Math on...
● Instagram: [ Ссылка ]
● Facebook: [ Ссылка ]
● Twitter: [ Ссылка ]
My Music Channel: [ Ссылка ]