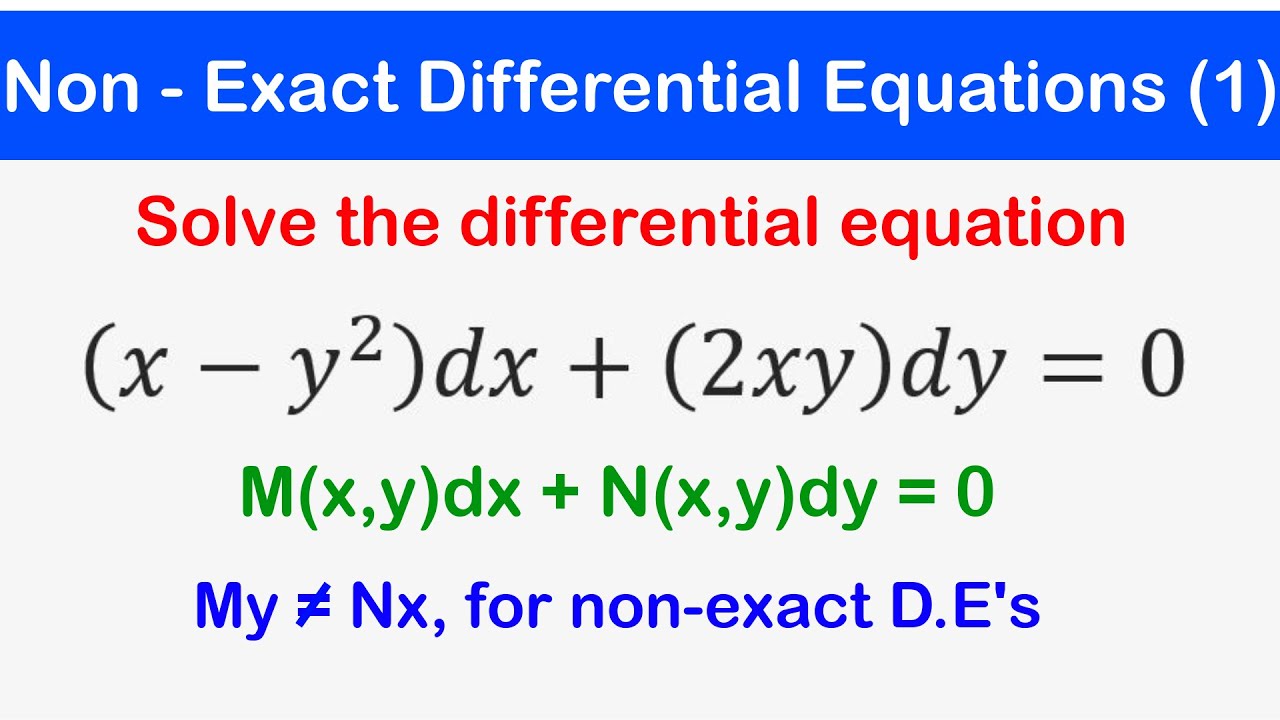
In this video, we shall learn how to transform a non-exact differential equation to an exact differential equation and solve for the general solution.
A differential equation of the form M(x,y)dx + N(x,y)dy = 0 is said to be non-exact if and only if the partial derivative of My is not = the partial derivative of Nx;
thus if
My is not = Nx.
A non exact differential equation can be made exact by multiplying the D.E by an integrating factor u(x) or U(y).
Ones the D.E is multiplied by the integrating factor, the differential equation becomes exact and hence the general solution can be found.
The solution of an exact differential equation must be of the form; f(x,y) = c, where c is a constant.
In this lesson we shall solve two major examples
Playlists on various Course
1. Applied Electricity
[ Ссылка ]
2. Linear Algebra / Math 151
[ Ссылка ]
3. Basic Mechanics
[ Ссылка ]
4. Calculus with Analysis / Calculus 1 / Math 152
[ Ссылка ]
5. Differential Equations / Math 251
[ Ссылка ]
6. Electric Circuit Theory / Circuit Design
[ Ссылка ]
Make sure to watch till the end.
Like, share, and subscribe.
Thank you.