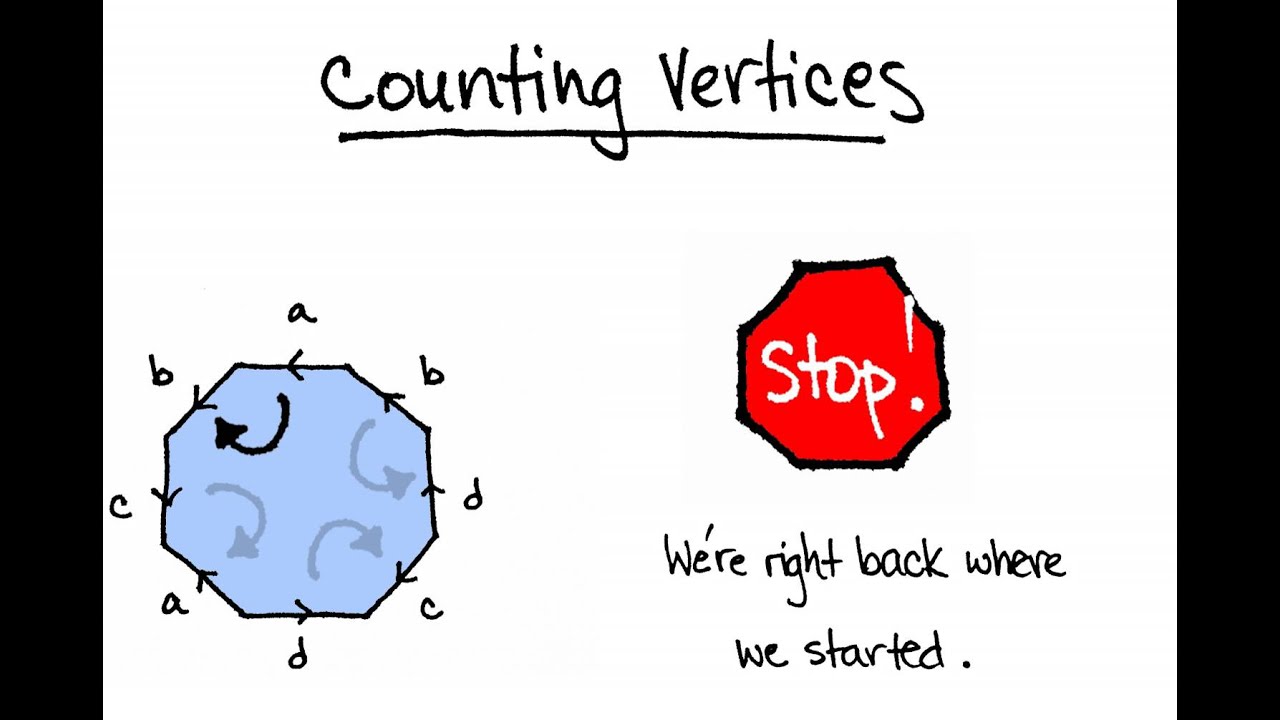
This video was created to accompany a blog post about the Classification Theorem of algebraic topology ([ Ссылка ]), and illustrates how to count the number of vertices in a polygon (a CW complex) in order to determine its Euler characteristic (= # vertices - # edges + # faces).
In short, the Classification Theorem states that every closed surface is homeomorphic to either a sphere, a connected sum of tori, or a connected sum of projective planes. In the video above, certain edges of an octagon are glued together according to the labeling indicated, and we wish to determine what surface it represents: a sphere, a connected sum of tori, or a connected sum of projective planes.
To do this, we must determine its Euler characteristic, its orientability, and its genus. This video clip demonstrates how to find the number of vertices our surface has, which will enable us to compute the Euler characteristic.
For more details, be sure to follow the link to the full blog post: [ Ссылка ]