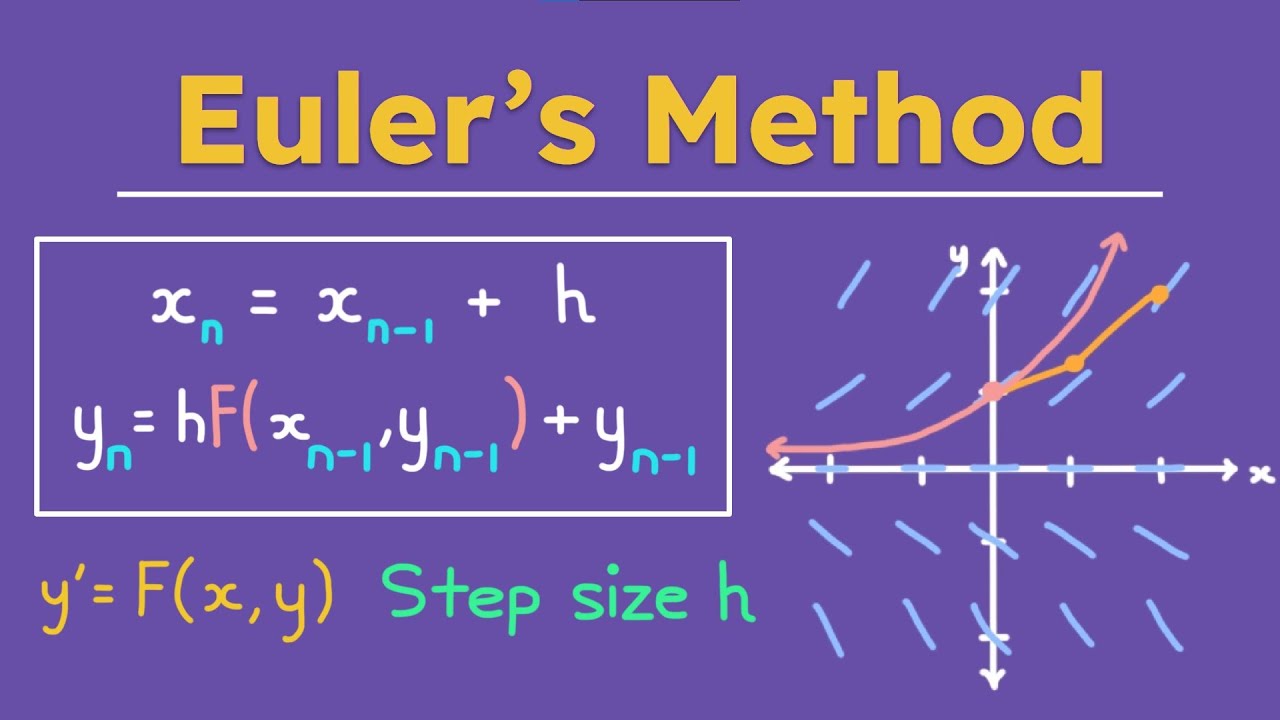
In this video, I will show you how to use Euler's Method to estimate the y value of differential equations. Euler's Method is quite simple as there is a formula that you just use over and over until you reach the point you need. You will also need to draw a slope field, also known as the direction field, which can be drawn from a differential equation y' = F(x,y). A differential equation is an equation with an unknown variables and its derivatives. You also need the piece of information about the initial condition or the initial value, which is basically the starting point of the differential equation and can be used to draw the solution curve. Euler's Method is an important topic in any Calculus 2 course, and there are many applications of Euler's method in real life.

We'll delve into the fascinating world of direction fields, also known as slope fields, in Calculus 2. Direction fields allow us to visualize the slopes of solutions to differential equations and gain a deeper understanding of how functions behave. Join us as we unravel the concepts behind direction fields, explore their applications, and demonstrate how to construct them with practical examples. Whether you're a student struggling to grasp slope fields or a math enthusiast eager to expand your knowledge, this video will guide you through the intricacies of direction fields and unlock new insights in Calculus 2.

Don't miss out on this enlightening exploration! Subscribe to our channel and hit the notification bell to stay updated with more captivating math content.

свернуть