We prove the limit law for a constant multiplied by a convergent sequence. If a_n converges to a and c is a real number, then the sequence c*a_n converges to c*a. As in, the constant multiple of a convergent sequence converges to its limit multiplied by the same constant. This is a straightforward proof using the epsilon definition of a convergent sequence, except for the case where c is 0. If c = 0, our result follows from the fact that a convergent sequence converges to its constant value.
Proof of Triangle Inequality Theorem: [ Ссылка ]
Proof of Reverse Triangle Inequality: [ Ссылка ]
Definition of the Limit of a Sequence: [ Ссылка ]
Proof that a Constant Sequence Converges to its Constant Value: [ Ссылка ]
Limit Law for Sum of Convergent Sequences: [ Ссылка ]
Limit Law for Difference of Convergent Sequences: [ Ссылка ]
Limit Law for Product of Convergent Sequences: [ Ссылка ]
Limit Law for Quotient of Convergent Sequences: [ Ссылка ]
Proving All the Sequence Limit Laws: [ Ссылка ]
Real Analysis Playlist: [ Ссылка ]
★DONATE★
◆ Support Wrath of Math on Patreon for early access to new videos and other exclusive benefits: [ Ссылка ]
◆ Donate on PayPal: [ Ссылка ]
Thanks to Robert Rennie and Barbara Sharrock for their generous support on Patreon!
Thanks to Crayon Angel, my favorite musician in the world, who upon my request gave me permission to use his music in my math lessons: [ Ссылка ]
Follow Wrath of Math on...
● Instagram: [ Ссылка ]
● Facebook: [ Ссылка ]
● Twitter: [ Ссылка ]
My Music Channel: [ Ссылка ]
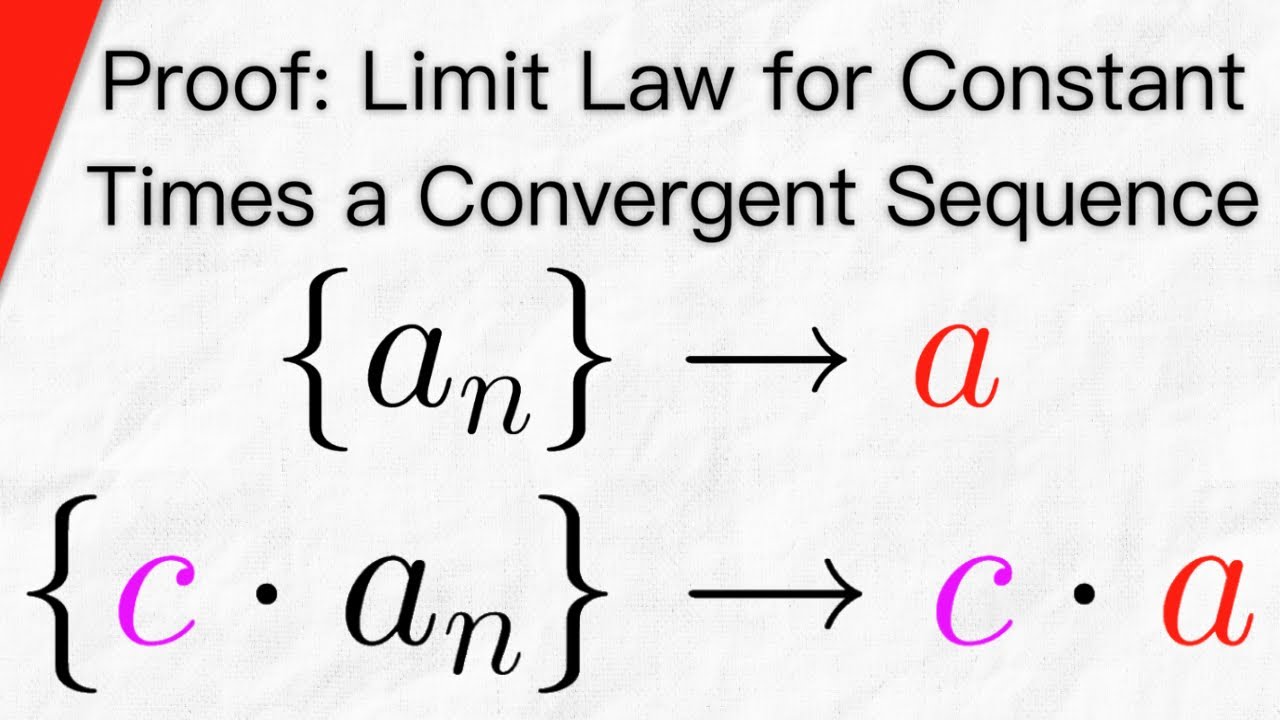



























































![How To Get High Ticket SMMA Clients [STEP-BY-STEP]](https://i.ytimg.com/vi/ah8HTKWyLEs/mqdefault.jpg)





![Тема 25. Звуки [з], [з’], буквы З, з](https://i.ytimg.com/vi/uF3DQTPxVSs/mqdefault.jpg)





