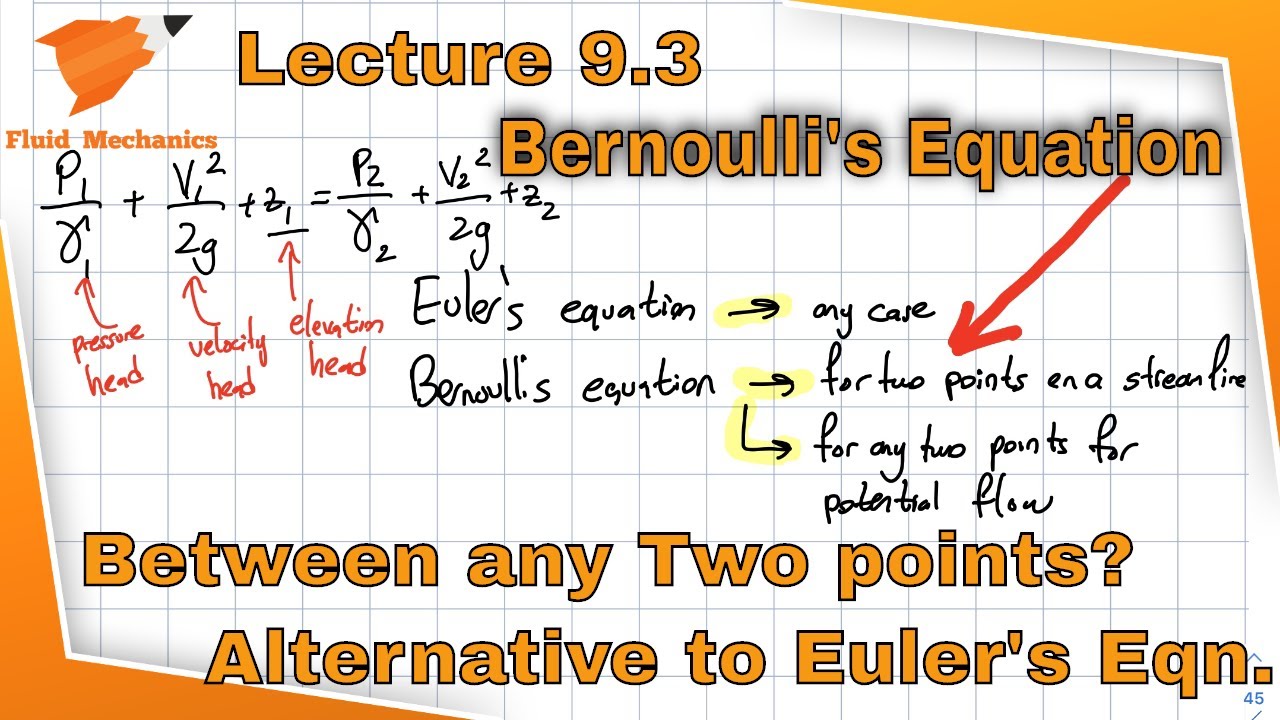
In this segment, we discuss Bernoulli’s equation as an alternative to Euler’s equations. We discuss the conditions that need to be satisfied as well as layout a three-option path for solving questions.
Module 9: The differential form of the Conservation of Momentum:
In module 6, we covered that the conservation of momentum can be derived from Newton's second law that states that the time rate of change of the linear momentum of the system = Sum of external forces acting on the system.
In module 9, we express this equation in the differential form, called the Euler's equations. We also introduce the alternative to Euler's equation for irrotational flow, Bernoulli's equation, which can be applied between any two points in the flow.
Student Learning Outcomes:
After completing this module, you should be able to:
1)Apply the Euler's and Bernoulli's (where applicable) equations to obtain pressure gradient and pressure differences between various points in the flow This material is based upon work supported by the National Science Foundation under Grant No. 2019664. Any opinions, findings, and conclusions, or recommendations expressed in this material are those of the author and do not necessarily reflect the views of the National Science Foundation.