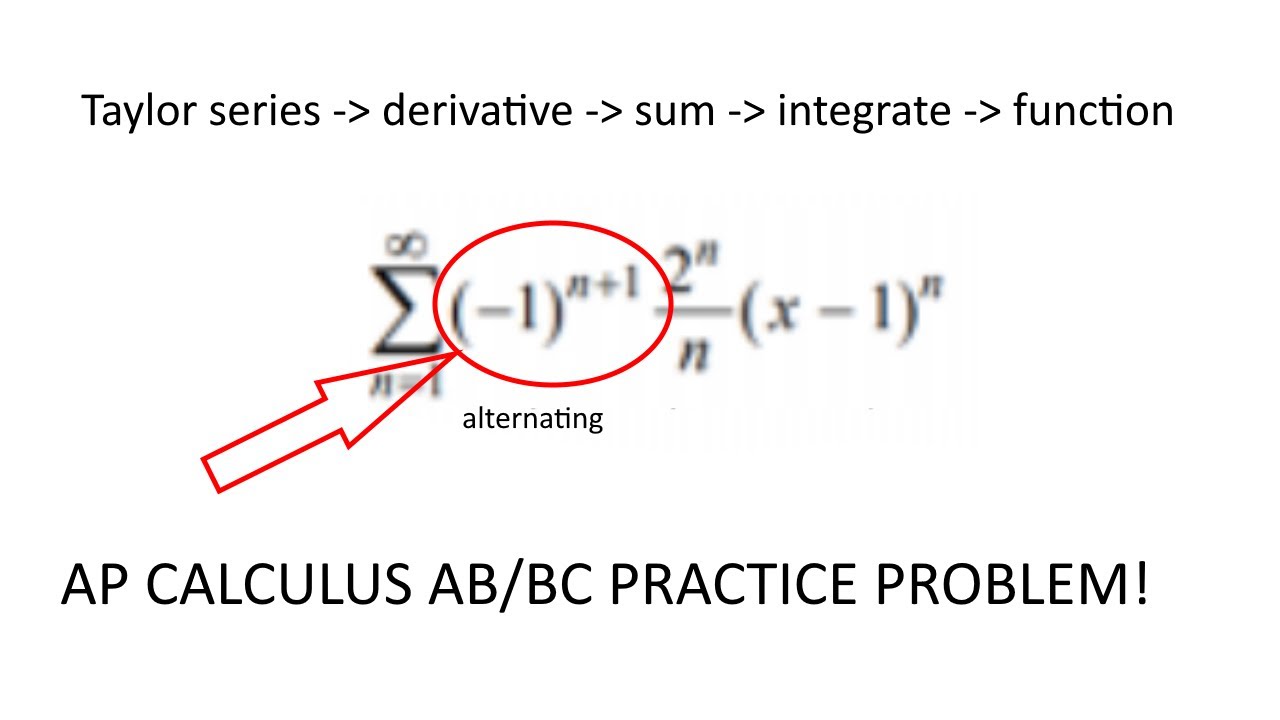
Welcome back guys. In today's video, I go over a problem that has you going back and forth between a function, a sum, a Taylor series, and a geometric series. This sounds complicated, but really these ideas are so closely related that going between them is simple! However, simple doesn't mean easy to understand necessarily, so if you have any questions please feel free to ask in the comments.

2x speed reminder

Important things:

Geometric series sum formula: You can derive this relatively easily if you need to, but remembering "a over 1 minus r" isn't too bad.

Radius of convergence: This goes hand in hand with the ratio test, which really you should already have memorized, as it's pretty useful. If you can do the ratio test, you can do this, especially when you don't even need to check the endpoints.

Derivative of a Taylor series: Remember: for a Taylor/MacLaurin series, it is almost always easy to differentiate and integrate term by term. This comes up especially when you have differential equations involving Taylor series.

свернуть