A surface is 2-dimensional, so we need two parameters (typically u and v) to parametrize it. Several examples of coming up with parametrizations r(u,v), plus the domains for u and v. Examples include polar coordinates. Multivariable Calculus Unit 3 Lecture 4.
The goal is to create descriptions suitable for calculus applications. Important note:
- Curves: Being one-dimensional, curves require only one parameter, typically denoted as 𝑡.
- Surfaces: As two-dimensional objects, surfaces require two parameters for a complete description. We usually use 𝑢 and 𝑣 as these parameters.
Examples of Surface Parametrization
1. Saddle Surface 𝑧=𝑥𝑦:
- Parametrization: 𝑟⃗ (𝑢,𝑣)=(𝑢,𝑣,𝑢𝑣), where 𝑢 and 𝑣 are real numbers.
- This parametrization captures the essence of the saddle surface, where 𝑧 is the product of 𝑥 and 𝑦.
Other examples include:
2. Upper Hemisphere (two ways)
3. Planes
4. Circular Cylinder 𝑥^2+𝑧^2=1 as 𝑟⃗ (𝑢,𝑣)=(cos(𝑢),𝑣,sin(𝑢)), with 𝑢 ranging from 0 to 2𝜋 and 𝑣 being any real number.
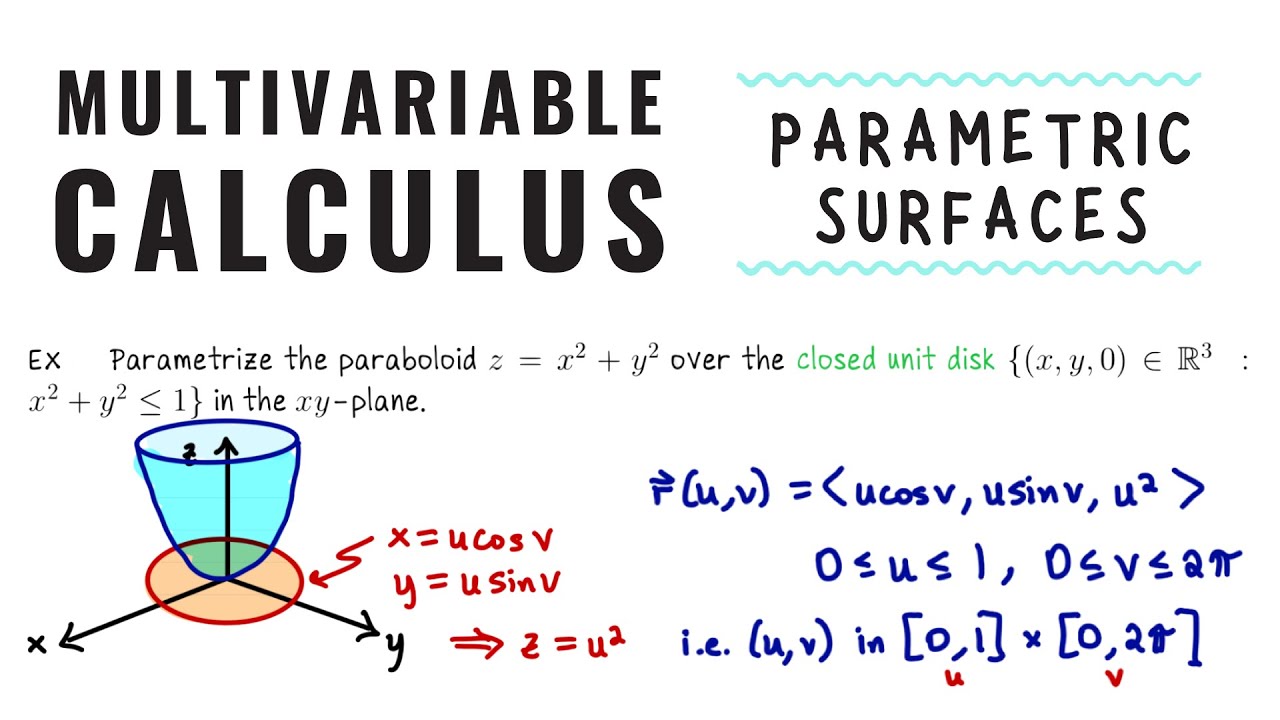
5. Paraboloid 𝑧=𝑥^2+𝑦^2 over a unit disc
#calculus #multivariablecalculus #mathematics #parametricsurfaces #iitjammathematics #calculus3