I plan to discuss three problems of extremal statistics in which unusual (but related to each other) features arise:
a) statistics of two-dimensional ”stretched” random walks above a semicircle,
b) spectral properties of sparse random matrices,
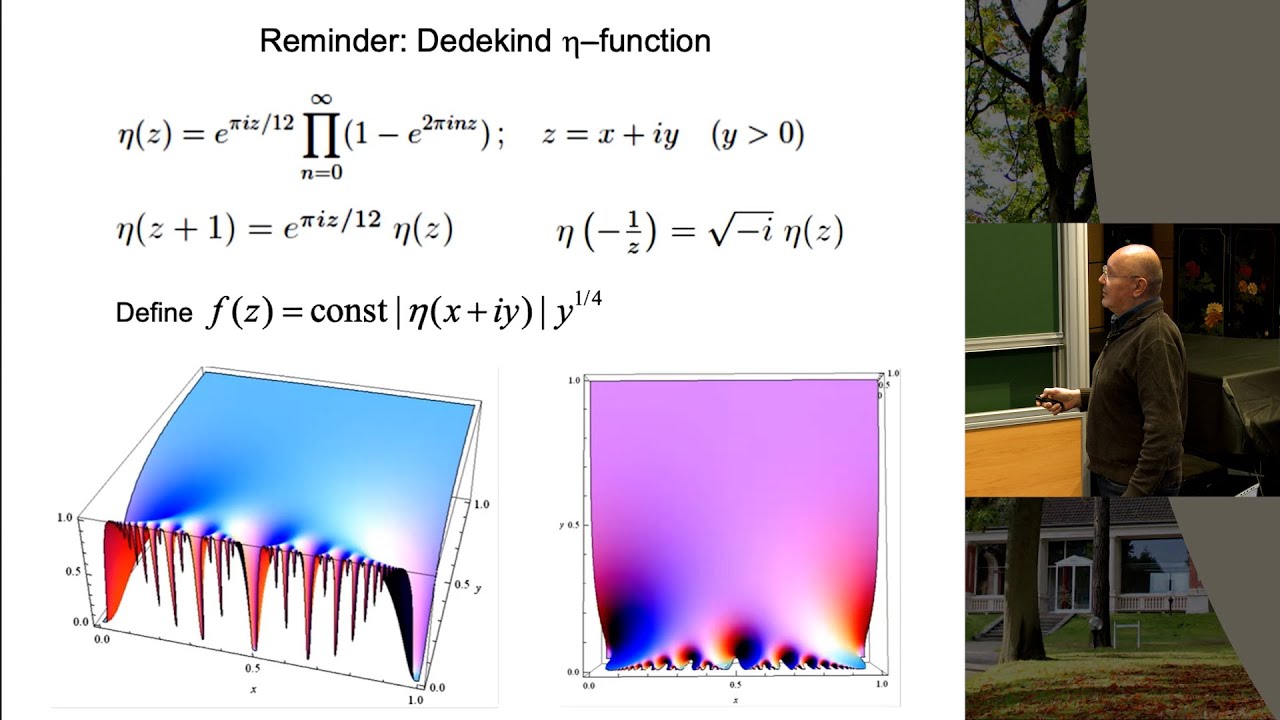
c) statistics of one-dimensional paths in the Poissonian field of traps. I will pay attention to the relationship of these problems with the Anderson localization in 1D, and with some number-theoretic properties of eta-Dedekind function.
Sergei Nechaev (Poncelet Laboratory Moscow)