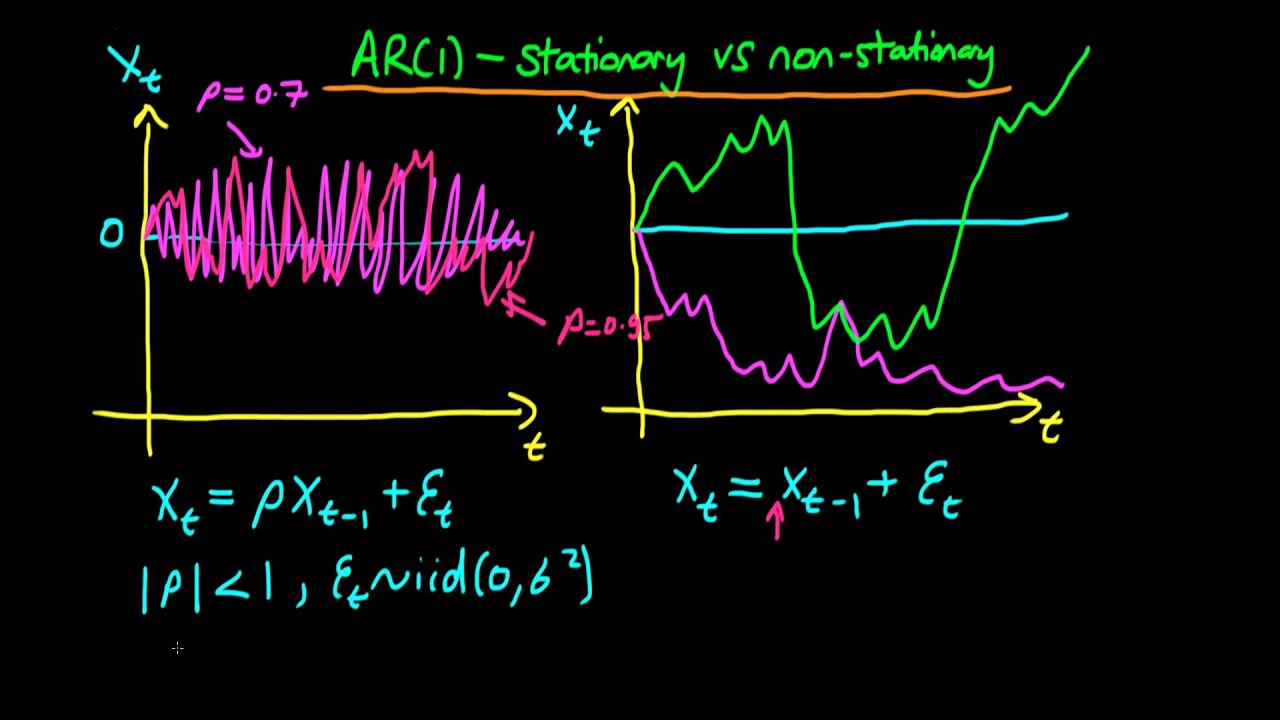
This video explains the qualitative difference between stationary and non-stationary AR(1) processes, and provides a simulation at the end in Matlab/Octave to demonstrate the difference.



clear; close all; clc;

n=10000; % Setting the number of time periods equal to 10000.
b=1;
rho=1; %This is the coefficient on the lagged part of x

x=zeros(n,1); % Initialise the vector x
x(1)=0;

for i = 2:n
x(i)=rho*x(i-1)+b*randn();
end

zoom=1.0;
FigHandle = figure('Position', [750, 300, 1049*zoom, 895*zoom]);
plot(x, 'LineWidth', 1.4)
ylabel('X(t)')
xlabel('t')


I also include the same in R (Courtesy of Jesse Maurais):

z = rnorm(1000)
gen = function(rho) {
x = numeric(length(z))
x[1] = z[1]
for (i in 2:length(z)) {
x[i] = rho*x[i-1] + z[i]
}
x
}
display = function(rho) {
x = gen(rho)
plot(x, main=as.character(rho))
lines(x)
}
for (it in 1:100) {
display(it/100)
Sys.sleep(0.5)
} Check out [ Ссылка ] for course materials, and information regarding updates on each of the courses. Quite excitingly (for me at least), I am about to publish a whole series of new videos on Bayesian statistics on youtube. See here for information: [ Ссылка ] Accompanying this series, there will be a book: [ Ссылка ]

свернуть