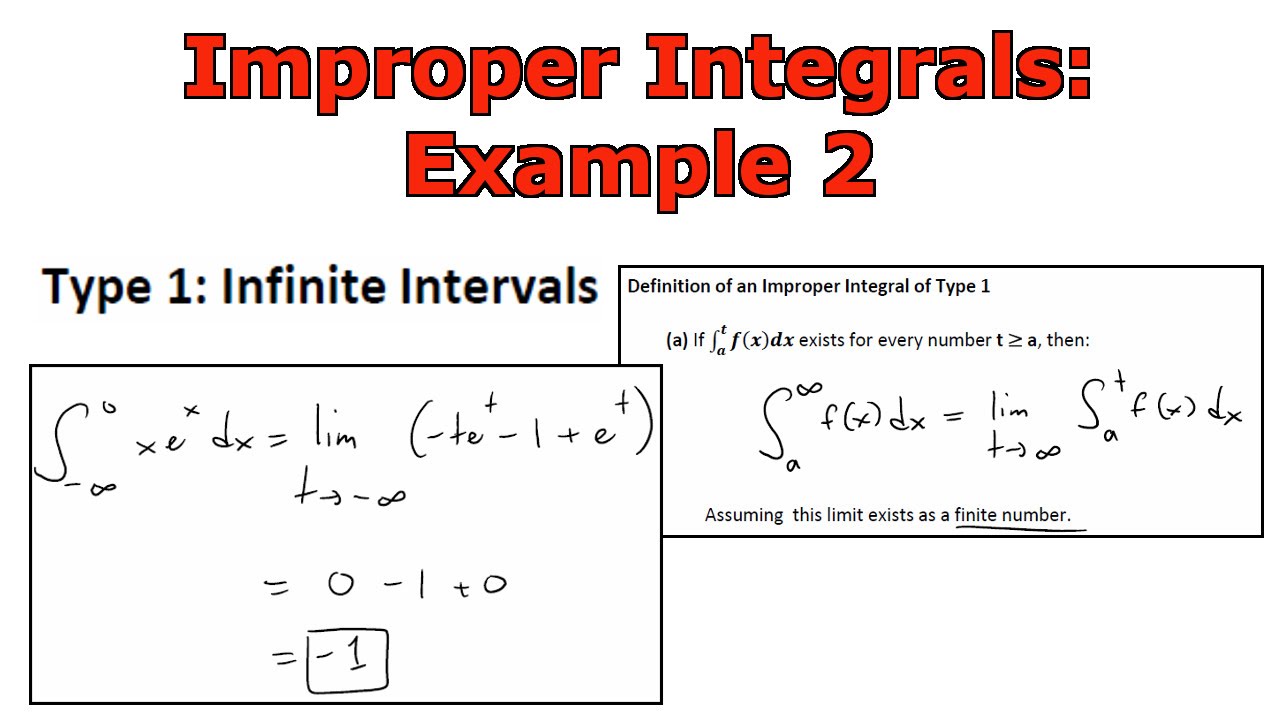
In this video I go over another example on improper integrals and this time solve the integral of the function x*e^x from x = -∞ to x = 0. In solving this improper integral, I had to use the method of integration by parts as well as the L'Hospital's Rule for evaluating the resulting limit and show that this function does in fact converge and the answer is simply -1.
Download the notes in my video: [ Ссылка ]
View Video Notes on Steemit: [ Ссылка ]
Related Videos:
Improper Integrals: Example 1: Divergent Sum: [ Ссылка ]
Improper Integrals: Introduction: [ Ссылка ]
Improper Integrals: Type 1: Infinite Intervals: [ Ссылка ]
Infinite Limits - Precise Definition: [ Ссылка ]
Infinite Limits - Vertical Asymptotes: [ Ссылка ]
L'Hospital's Rule - A general Proof: [ Ссылка ]
Integration by Parts: Proof: [ Ссылка ]
Derivative of y = a^x and e^x: Using the Chain Rule: [ Ссылка ]
Power Functions Part 2: What is x^0 and 0^0???: [ Ссылка ] .
------------------------------------------------------
Become a MES Super Fan! [ Ссылка ]
DONATE! ʕ •ᴥ•ʔ [ Ссылка ]
SUBSCRIBE via EMAIL: [ Ссылка ]
MES Links: [ Ссылка ]
MES Truth: [ Ссылка ]
Official Website: [ Ссылка ]
Hive: [ Ссылка ]
Email me: contact@mes.fm
Free Calculators: [ Ссылка ]
BMI Calculator: [ Ссылка ]
Grade Calculator: [ Ссылка ]
Mortgage Calculator: [ Ссылка ]
Percentage Calculator: [ Ссылка ]
Free Online Tools: [ Ссылка ]
iPhone and Android Apps: [ Ссылка ]
Improper Integrals: Example 2: x*e^x
Теги
matheasymath easyeasymatheasy mathmathonline calculatorcalculatorfree calculatoronline toolsfree online toolsintegralsintegral calculusmath exampleintegral exampleimproper integralsimproper integral exampleinfinite limitsinfinite integralsintegrals as limitsl'hospital's ruleintegration by partsexample 2